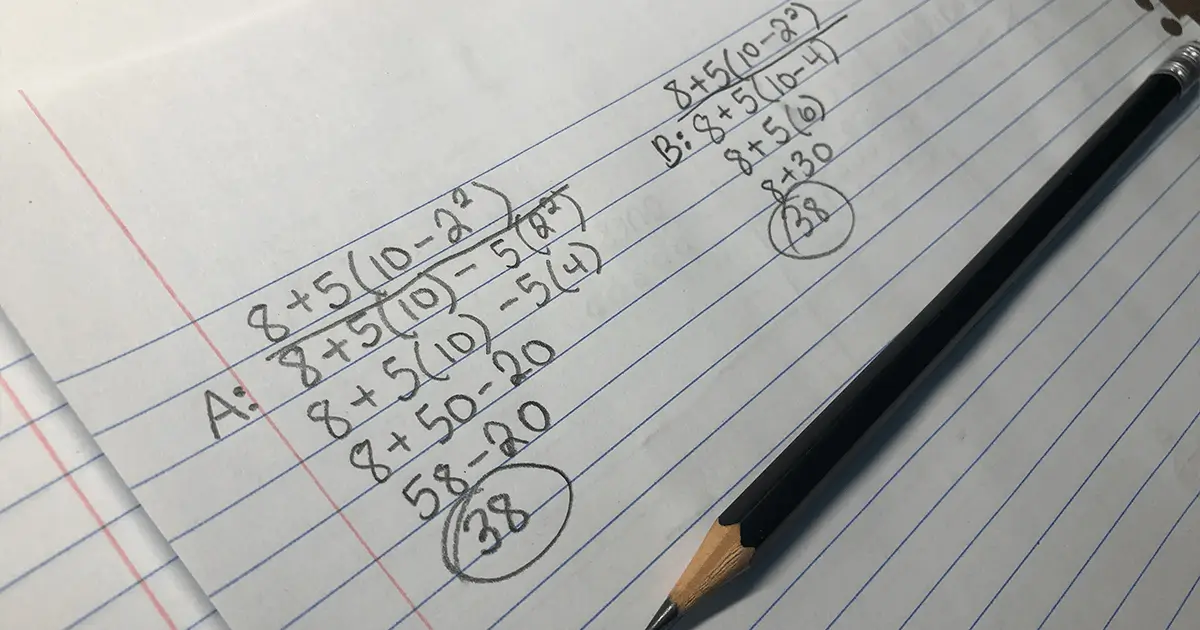Teaching Order of Operations Through the Relationships of the Operations

STRATEGY

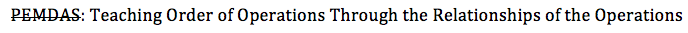
Not long ago, if someone asked me to teach my students how to evaluate this expression, 15+ (9–4)- 2³, I would have immediately thought of teaching them how to use PEMDAS. However, what if they learned the reason behind this mnemonic by studying the relationship of the operations?

Why is it important to follow a set of rules in math? Let’s take a look.

By looking at the student work above it’s clear Jessie and Matt both had a strategy, but who is correct? Just like everyone drives on the right side of the road in the United States of America, consistent conventions ensure everyone is following the same process and arrives at the same answer.

Grade 6 Module 4 Lesson 6 focuses on the use of order of operations to evaluate numerical expressions. However, a quick skim of this lesson will undoubtedly inform you that this is not the traditional approach. So, let’s dig in and find out more!

In Lesson 3 of this module, students strengthen their understanding of the relationship between multiplication and addition. They explore and evaluate identities such as 3 x g = g + g + g. Given that each unit in the tape diagram represents one, notice how the first tape diagram represents the expression 2 + 2 + 2 + 2 and the second tape diagram represents the expression 4 x 2

Students notice that each model has the same number of units, and through intentional questioning, they conclude the values of the expressions are equivalent. After another example and a discussion of the relationship between 3 x 4 and 4 + 4 + 4, students explore the identity 3g = g + g + g. Students conclude that no matter what number replaces the variable, g, after adding g three times, the value is the same as multiplying g by three. By building the tape diagrams prior to this identity, students are able to visualize this relationship between repeated addition and multiplication (which is often a more efficient method than repeatedly adding the same number)

In Lesson 4, students discover the relationship of division and subtraction. In the example below, students determine that the number of times the divisor (x) is subtracted from the dividend (20) is the same number as the quotient (5). We determine in this example that the quotient is 5 because there are 5 equal groups of the same number being subtracted. The quotient is telling us how many times we’re subtracting the divisor from the dividend

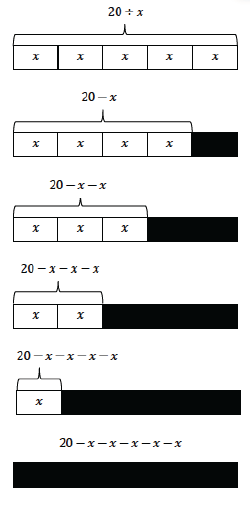
In this diagram, we can also see that the expression 20 ÷ x means that the number that x represents can be subtracted exactly 5 times from 20. The subtraction equation 20–4–4–4–4–4=0 tells us 4 can be subtracted exactly 5 times from 20 before there is no remainder, so the divisor in this expression is 4. With the model and the work in this lesson, students explore the relationship between repeated subtraction and division (which is often a more efficient method when repeatedly subtracting the same number)

Students also tie together the relationship between all four operations at the end of Lesson 4. These connections are critical as they use these relationships to evaluate expressions using the order of operations. Take a look at the image below.

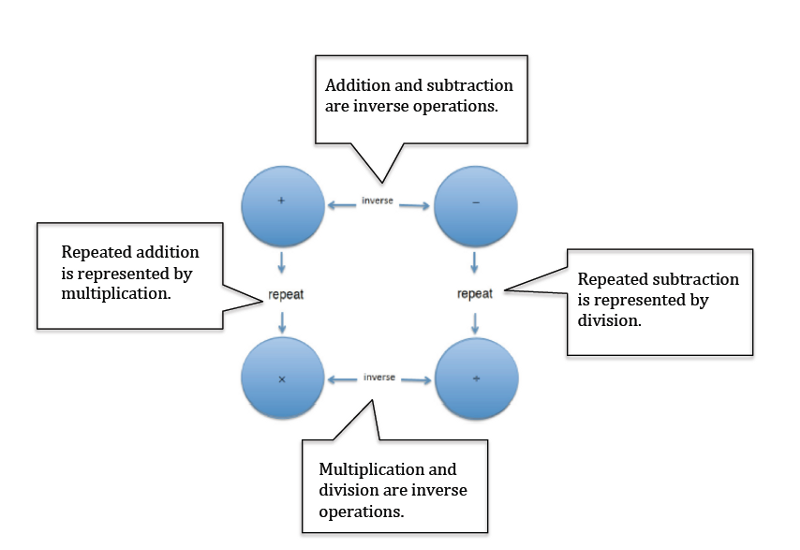
In A Story of Units, students learned that addition is a “shortcut” to counting on and subtraction is a “shortcut” to counting back. These operations are traditionally taught prior to other operations, and are ultimately evaluated last in an expression because they are the least complicated. In this blog, we’ve shown that multiplication and division are often more efficient ways to repeatedly add or subtract when the addends or subtrahends are the same. Therefore, multiplication and division are next in the sequence of learning the four operations, and are evaluated prior to addition and subtraction in an expression. It is apparent that the traditional order for performing certain operations was developed based on the complexity of the operation

So far, if the expression has only the operations of multiplication, division, addition and subtraction, then multiplication and division are evaluated first, and because they are inverse operations are equally powerful, they are evaluated from left to right. Addition and subtraction are evaluated next, also from left to right because they are inverse operations and hold the same power.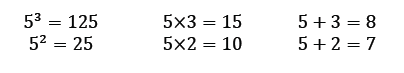

The amount of decrease in the first example of exponentiation is 100 and is much greater than the amount of decrease in the multiplication example (5) and the addition example (1). It’s clear how the repeated multiplication evident in the first example with exponents yields a greater decrease than the repeated addition seen in the second example and the basic addition of the last example.

In summary, you can take a look at how to evaluate a more complex expression in this video the Eureka Math Teach Eureka video series (clip 1:02–1:04)

But what happens to the order of operations when grouping symbols are introduced? Grouping symbols alert us that we may be doing things out of the typical order. With any problem, it’s context that prescribes how the problem should be solved.

Let’s take a look at the context below from Lesson 6.

The expression 4x5 +2 represents this context and tells us there are 4 groups of 5 since there are 4 people and the cost in dollars of each ticket can be represented by the number, 5 The additional price of the soft drink is represented by 2 because the cost of each soft drink, in dollars, is 2. A model for this scenario is below.

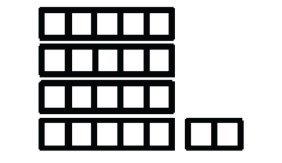
Now let’s look at another situation. 

How did the expression change to represent this new situation? Now, the total price per person changed from the price of one ticket (represented by the number 5) to the price of one ticket and a drink (represented by 5 + 2 or 7). So this expression is 4x(5+2) and tells us there are 4 groups of (5 + 2). Here is a model for this scenario.

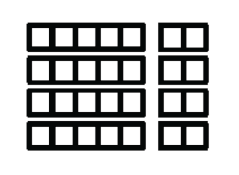
The value of this expression is 4x7 or (4 x 5) + (4 x 2), which is 28. Notice the importance of indicating how the numbers in the expression are grouped. Grouping symbols, such as the parentheses used in this problem, instruct us to evaluate operations involving parentheses first. 

You may have noticed Example A applied the distributive property to the second part of the expression, 5(10–2²), when evaluating the expression and Example B evaluated inside the parentheses (10–2²) first. In each example, the resulting value is the same but the way in which the students arrived at that solution was different. In these examples, it is evident that students do not always have to perform the operations within parentheses first, but more so the operations involving parentheses. This emphasizes the importance of encouraging students to compute in a manner that is most efficient to them

What we hope you walk away from this blog is just this: Instead of memorizing a mneumonic, such as PEMDAS, exploration of the relationships of operations builds the understanding we strive for and, ultimately, students become proficient mathematicians.

Up for a challenge? Take a look at the expression below. Can you evaluate the expression by applying the distributive property (similar to Example A above) and then by evaluating inside the parentheses first (similar to Example B above)? Which method works best for you?

3(2 + 8²) — 14

This blog is by Eureka Math writers Dawn Pensack and Erika Silva.